Soru Sor sayfası kullanılarak Türev konusu altında Üniversite seviyesi Türev Soruları ile ilgili sitemize gönderilen ve cevaplanan soruları içermektedir. Bu soru tipine ait soruları ve yaptığımız detaylı çözümleri aşağıda inceleyebilirsiniz. Yardımcı olması dileğiyle, iyi çalışmalar…
1.SORU
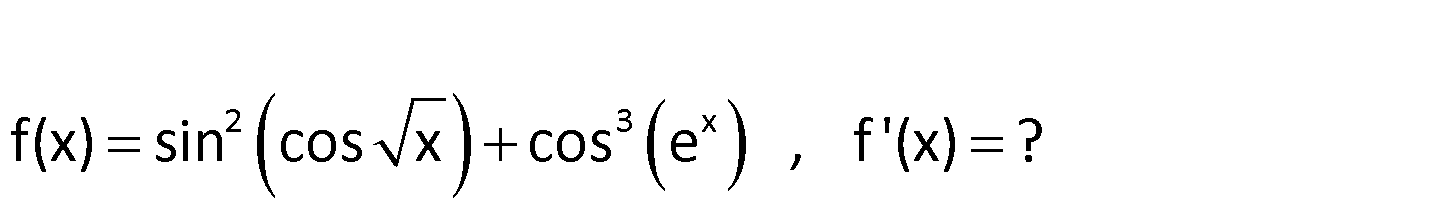

2.SORU
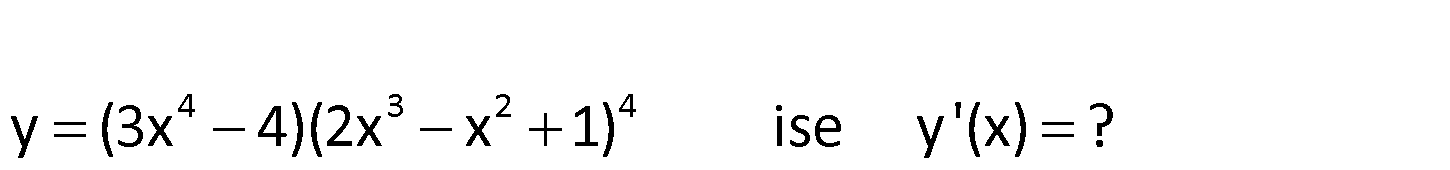
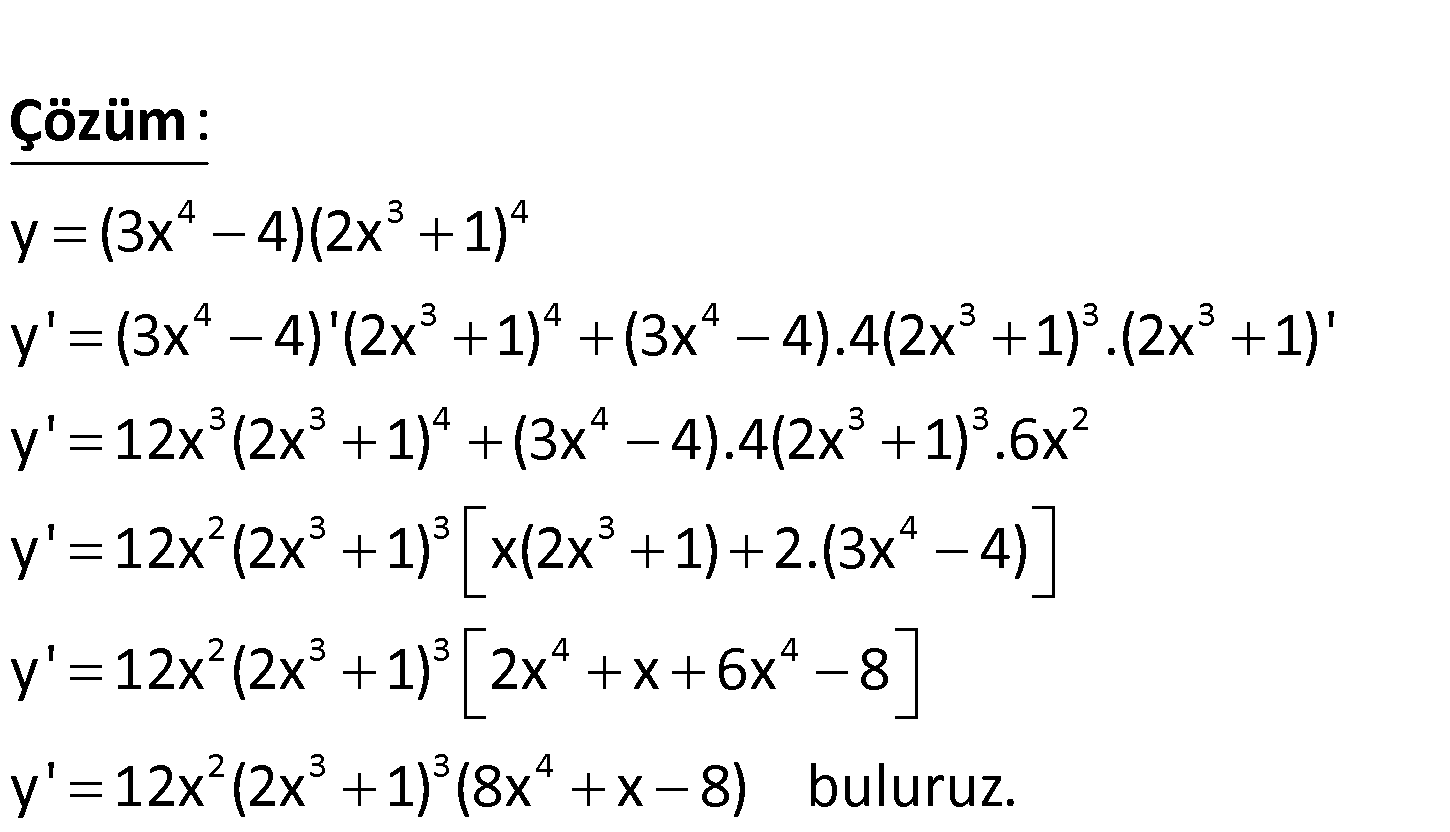
3.SORU
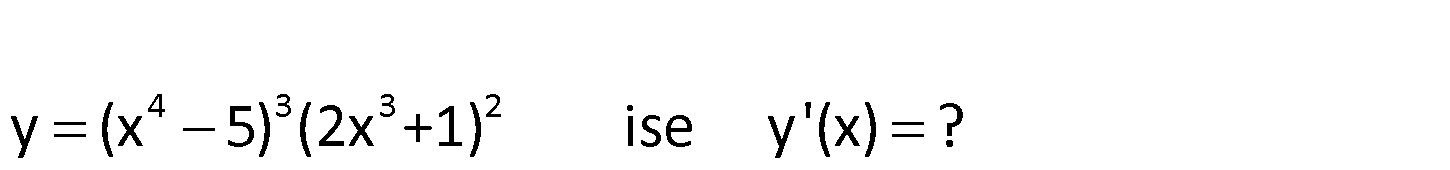
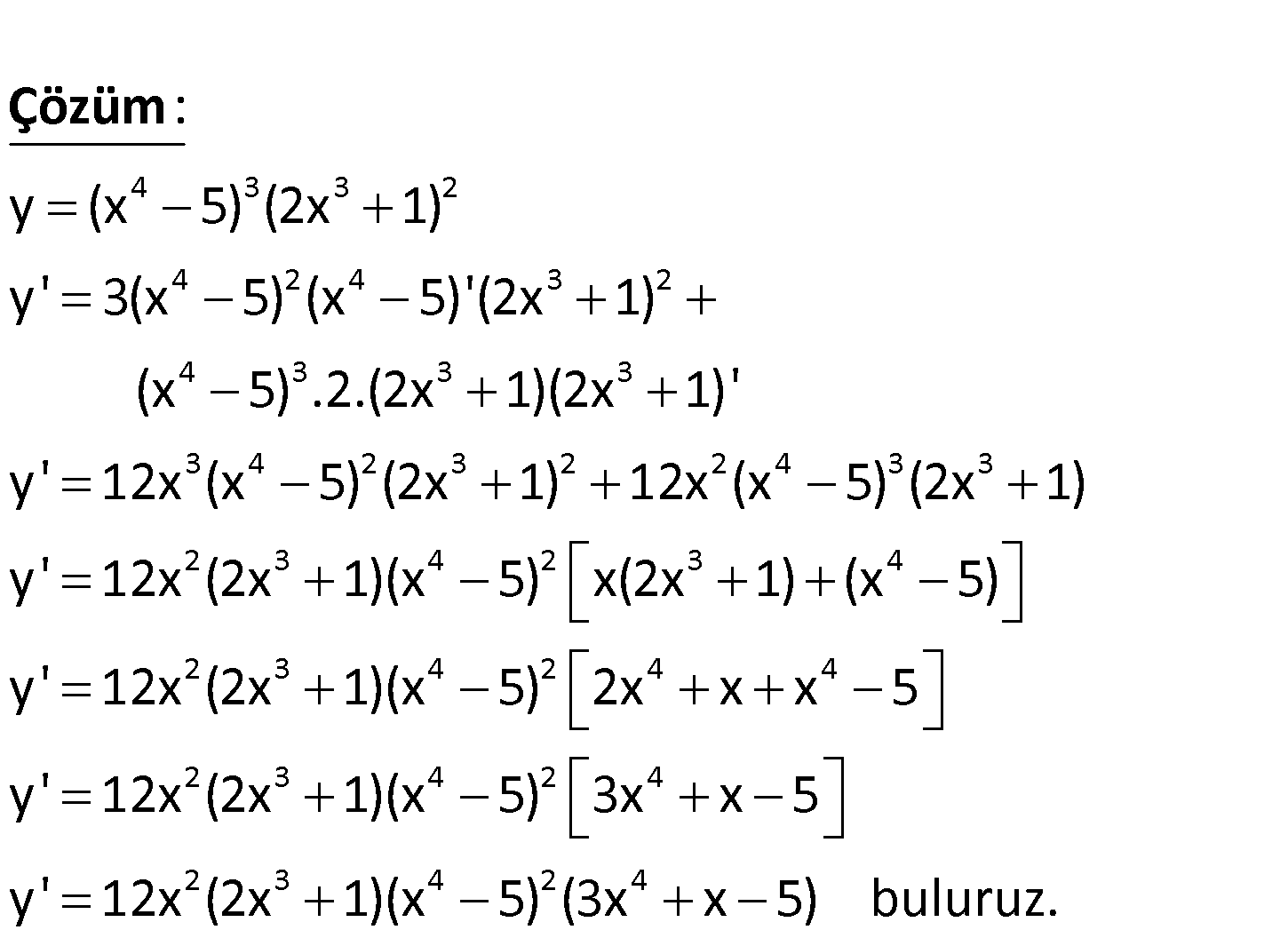
4.SORU
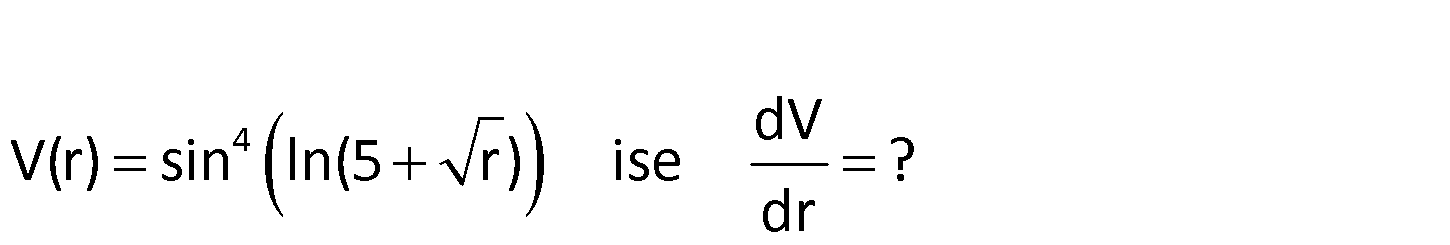

5.SORU
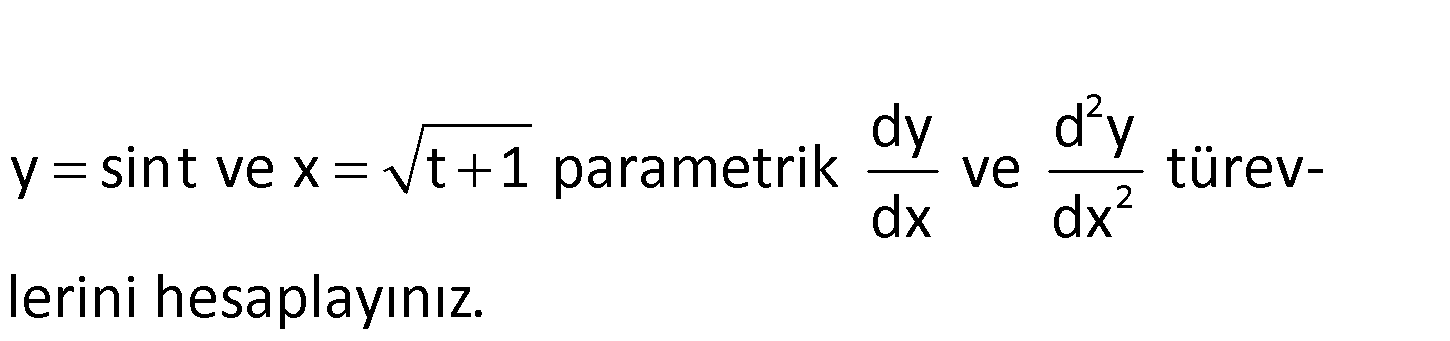
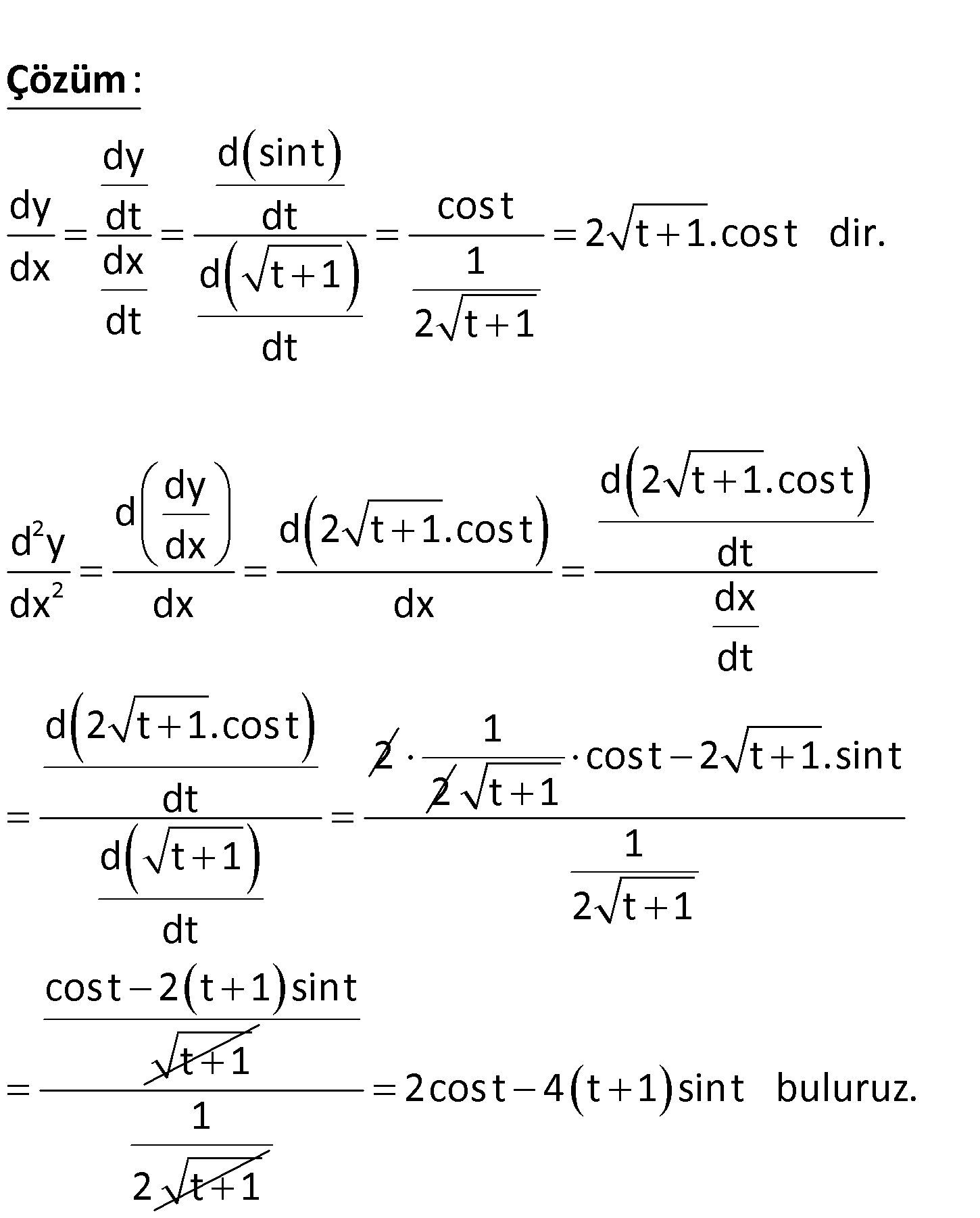
6.SORU
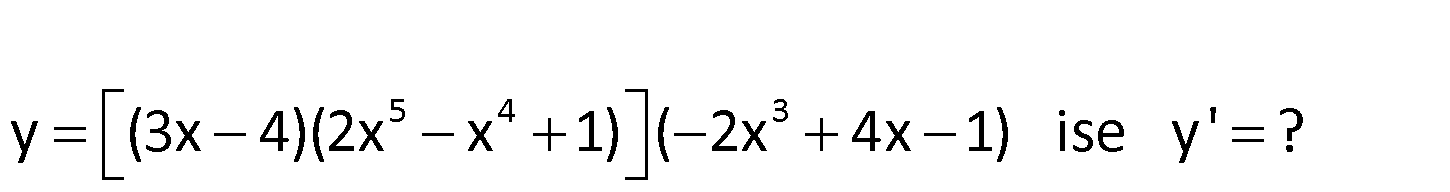
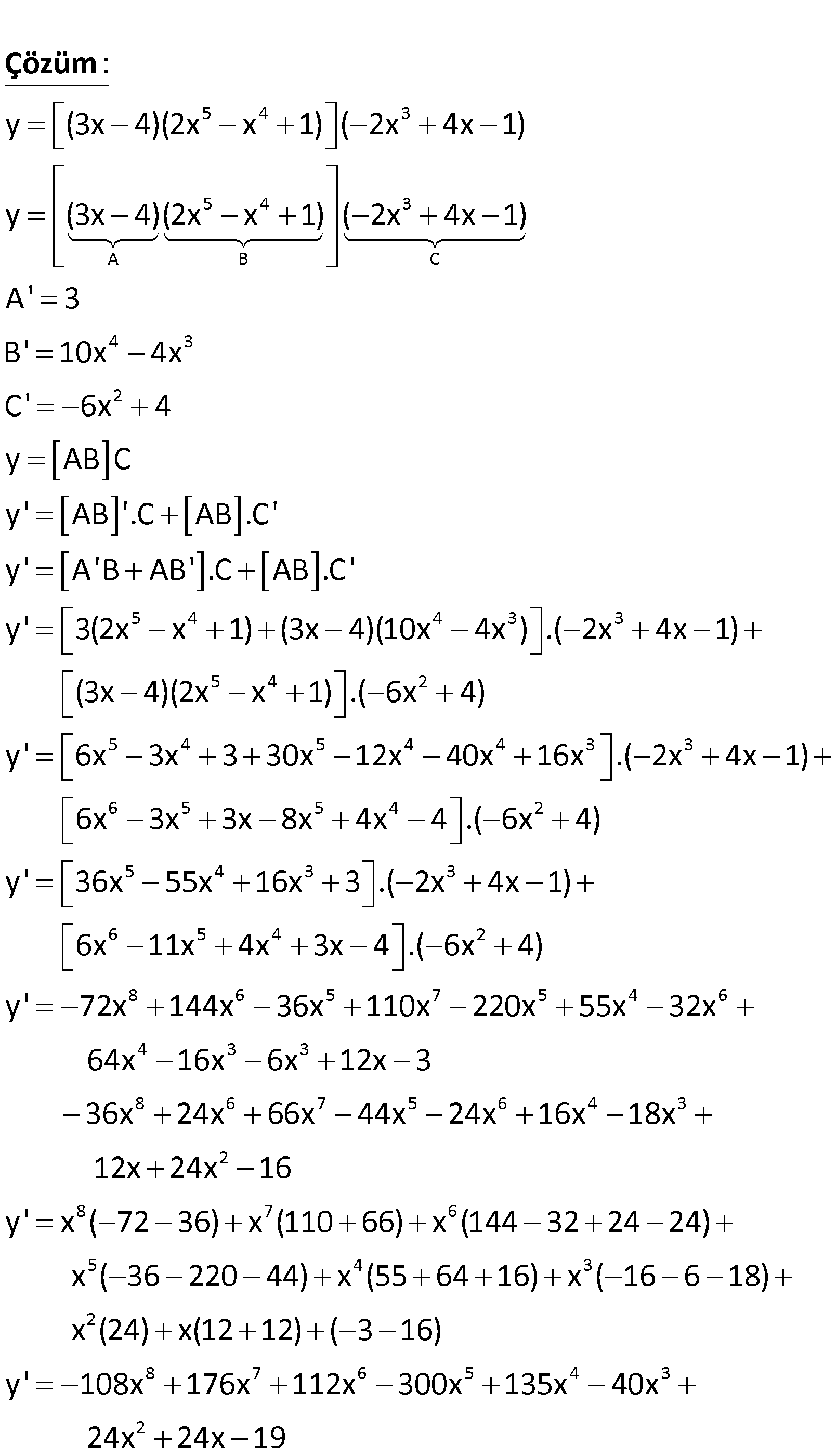
7.SORU
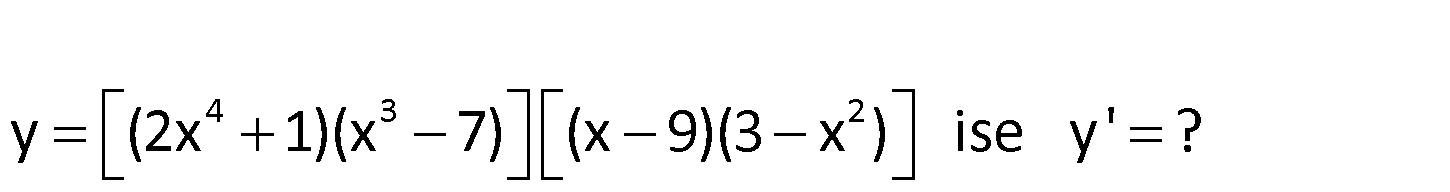

8.SORU


9.SORU
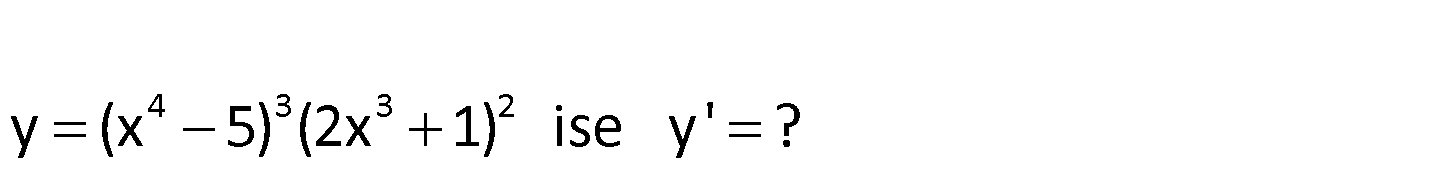
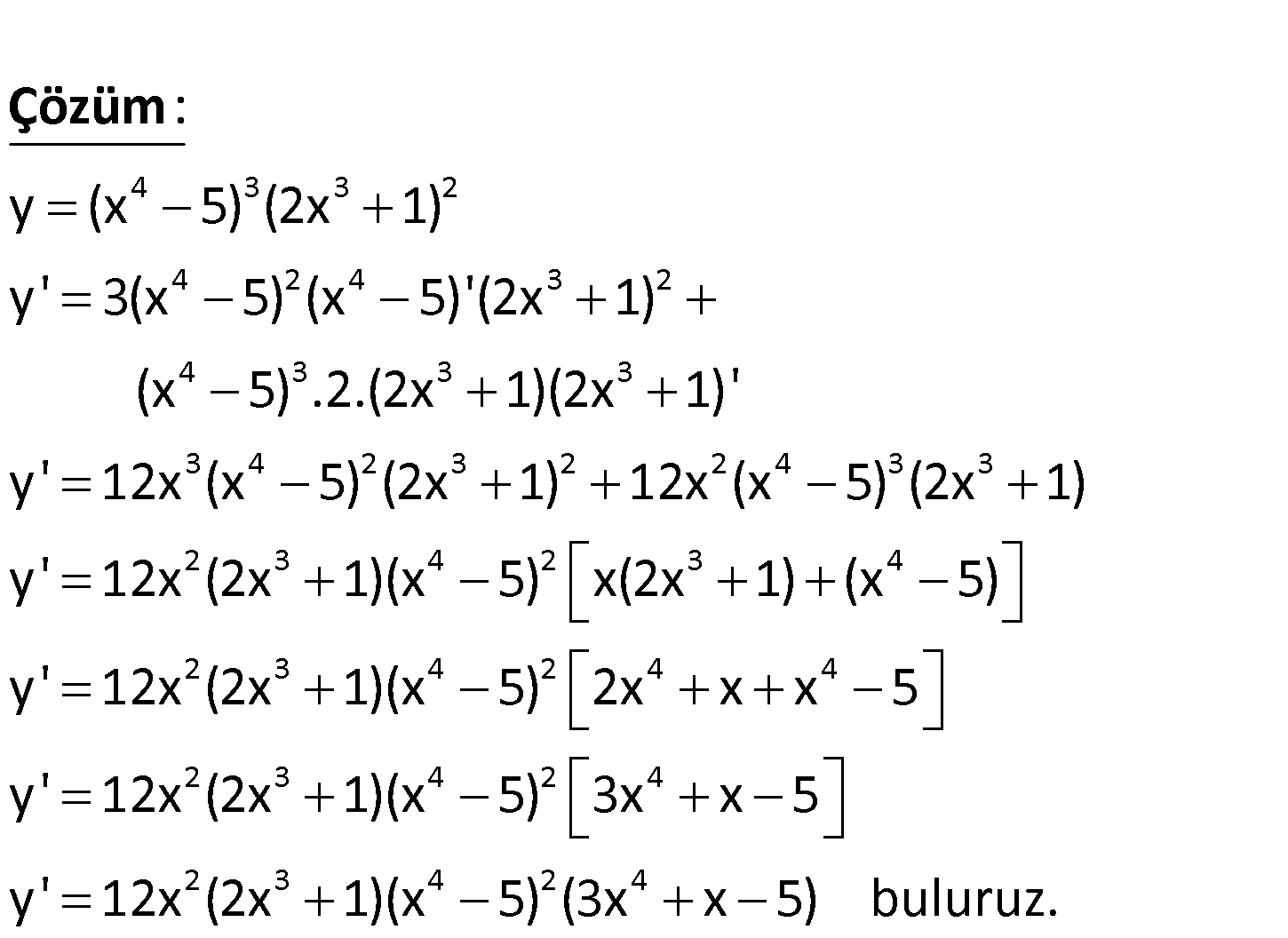
10.SORU


11.SORU
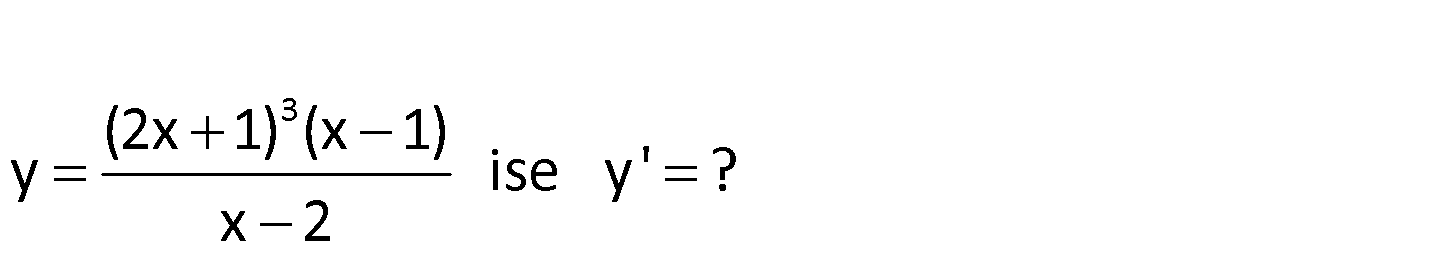
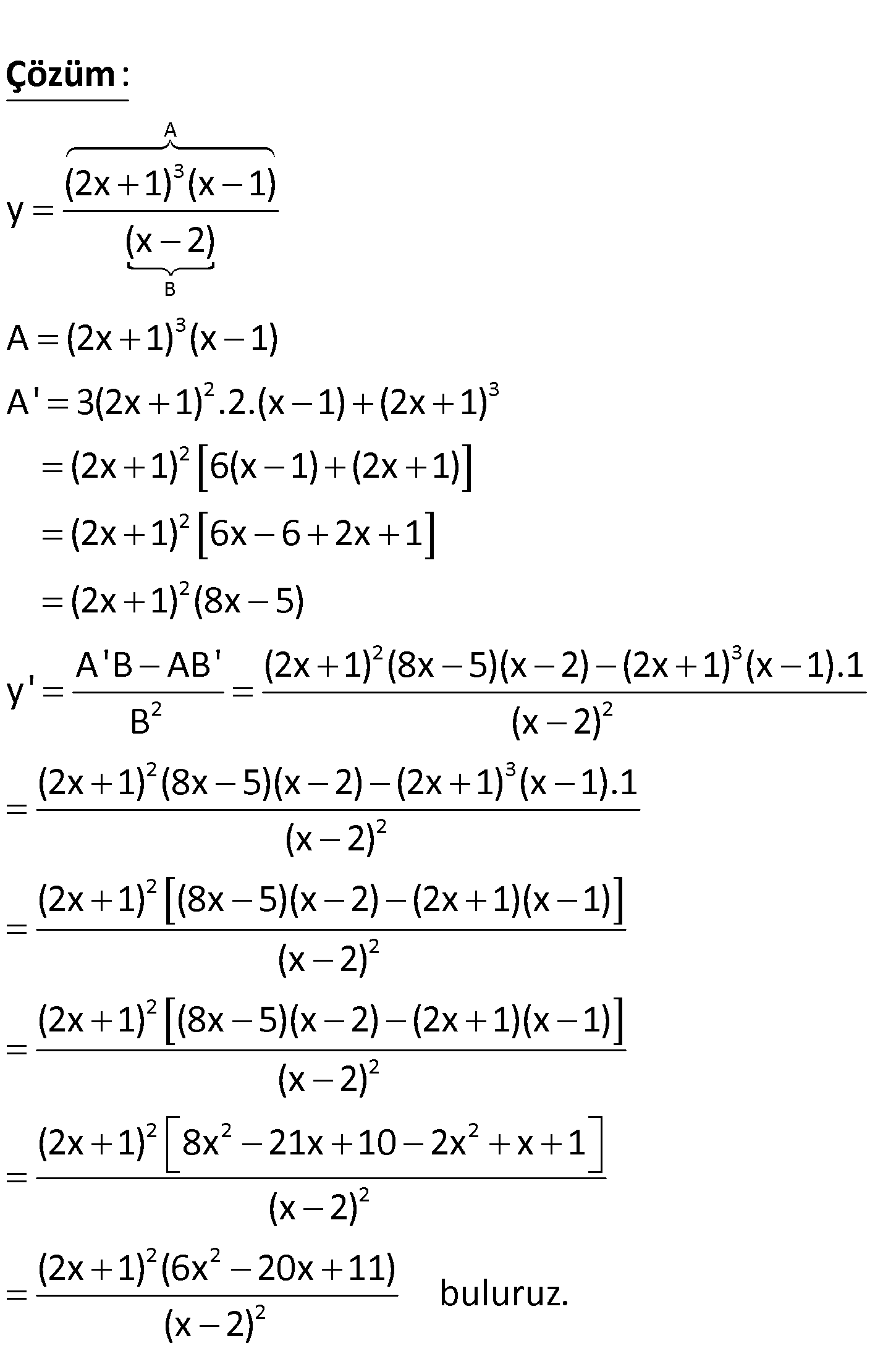
Diğer Soru Tipleri için Tıklayınız.
Not: Bu sayfadaki sorular, ziyaretçilerimiz tarafından gönderilmiştir. Telif hakkını ihlal eden durumlar için lütfen iletişim sayfasından bize bunları bildiriniz. Kısa süre içerisinde sitemizden bu sorular kaldırılacaktır.
Telif: Çözümler, sitemiz tarafından hazırlanmış olup izinsiz yayınlanıp, çoğaltılması yasaktır.
2 3 x f(x) sin cos x cos e , f ‘(x) ? www.matematikkolay.net 2 3 x x 2 x f(x) sin (cos x) cos (e ) f ‘(x) 2sin(cos x) sin(cos x) ‘ cos(e ) ‘3cos (e ) f ‘(x) 2sin(cos x).(cos x)’ : Çözüm x x 2 x x x x x x .cos(cos x) e ‘ sin(e ) 3cos (e f ‘(x) 2sin(cos x).( x)'( sin x).cos(cos x) e . sin(e ) 3cos 1 f ‘(x) 2sin(cos x) ( sin x).cos(cos x) e . sin(e ) 3cos 2 x 1 f ‘(x) ( sin x).2sin(cos x).cos(cos x) e . sin(e ) 3c 2 x x x 2 x os sin( x).sin(2cos x) f ‘(x) 3e .sin(e )cos (e ) buluruz. 2 x 48
www.matematikkolay.net 4 3 2 4 y (3x 4)(2x x 1) ise y'(x) ? 4 3 4 4 3 4 4 3 3 3 3 3 4 4 3 y (3x 4)(2x 1) y’ (3x 4)'(2x 1) (3x 4).4(2x 1) .(2x 1)’ y’ 12x (2x 1) (3x 4).4(2x : Çözüm 3 2 2 3 3 3 4 2 3 3 4 4 2 3 3 4 1) .6x y’ 12x (2x 1) x(2x 1) 2.(3x 4) y’ 12x (2x 1) 2x x 6x 8 y’ 12x (2x 1) (8x x 8) buluruz. 71
4 3 3 2 y (x 5) (2x +1) ise y'(x) ? 4 3 3 2 4 2 4 3 2 4 3 3 3 3 4 y (x 5) (2x 1) y’ 3(x 5) (x 5)'(2x 1) (x 5) .2.(2x 1)(2x 1)’ y’ 12x (x 5 : ) Çözüm 2 3 2 2 4 3 3 2 3 4 2 3 4 2 3 4 2 4 4 2 3 4 2 4 2 3 4 2 4 (2x 1) 12x (x 5) (2x 1) y’ 12x (2x 1)(x 5) x(2x 1) (x 5) y’ 12x (2x 1)(x 5) 2x x x 5 y’ 12x (2x 1)(x 5) 3x x 5 y’ 12x (2x 1)(x 5) (3x x 5) buluruz. 72
4 dV V(r) sin ln(5 r) ise ? dr 4 3 3 3 sin ln 5 r ifadesinin türevi 4sin ln 5 r sin ln 5 r ‘ 4sin ln 5 r cos ln 5 r ln 5 r ‘ 4sin ln 5 co ln : r s Çözüm 3 3 3 5 r ‘ 5 r 5 r 1 4sin ln 5 r cos ln 5 r 2 r 5 r 1 4sin ln 5 r cos ln 5 r 2 r 5 r 2sin ln 5 r .cos ln 5 r buluruz. 5 r r 102
2 2 dy d y y sint ve x t 1 parametrik ve türevdx dx lerini hesaplayınız. www.matematikkolay.net 2 2 dy d sint dy dt dt cos t 2 t 1.cos t dir. dx dx d t 1 1 dt 2 t 1 dt dy d 2 t d d 2 t 1.cos t d y dx dx dx dx : Çözüm 1.cos t dt dx dt d 2 t 1.cos t 2 dt d t 1 dt 1 2 cos t 2 t 1.sint t 1 1 2 t 1 cos t 2 t 1 sint t 1 1 2 t 1 2cos t 4t 1sint buluruz. 142
www.matematikkolay.net 5 4 3 y (3x 4)(2x x 1)(2x 4x 1) ise y’ ? 5 4 3 5 4 3 A B C y (3x 4)(2x x 1) ( 2x 4x 1) y (3x 4)(2x x 1) ( 2x 4x 1) : A’ 3 Çözüm 4 3 2 5 4 4 3 3 5 4 2 5 4 5 4 4 3 3 6 B’ 10x 4x C’ 6x 4 y AB C y’ AB ‘.C AB .C’ y’ A’B AB’ .C AB .C’ y’ 3(2x x 1) (3x 4)(10x 4x ) .( 2x 4x 1) (3x 4)(2x x 1) .( 6x 4) y’ 6x 3x 3 30x 12x 40x 16x .( 2x 4x 1) 6x 5 5 4 2 5 4 3 3 6 5 4 2 8 6 5 7 5 4 6 4 3 3 8 6 7 3x 3x 8x 4x 4 .( 6x 4) y’ 36x 55x 16x 3 .( 2x 4x 1) 6x 11x 4x 3x 4 .( 6x 4) y’ 72x 144x 36x 110x 220x 55x 32x 64x 16x 6x 12x 3 36x 24x 66x 44 5 6 4 3 2 8 7 6 5 4 3 2 8 7 6 5 4 x 24x 16x 18x 12x 24x 16 y’ x ( 72 36) x (110 66) x (144 32 24 24) x ( 36 220 44) x (55 64 16) x ( 16 6 18) x (24) x(12 12) ( 3 16) y’ 108x 176x 112x 300x 135x 4 3 2 0x 24x 24x 19 145
www.matematikkolay.net 4 3 2 y (2x 1)(x 7) (x 9)(3x ) ise y’ ? 4 3 2 A B 4 3 7 4 3 y (2x 1)(x 7) (x 9)(3 x ) A (2x 1)(x 7) 2x 14x x 7 A’ 14 : Çözüm 6 3 2 2 3 2 2 6 3 2 3 2 9 8 7 6 6 5 4 3 5 4 3 2 9 8 7 6 5 x 56x 3x B (x 9)(3 x ) x 9x 3x 27 B’ 3x 18x 3 y’ A’B AB’ A’B (14x 56x 3x )( x 9x 3x 27) 14x 126x 42x 378x 56x 504x 168x 1512x 3x 27x 9x 81x 14x 126x 42x 322x 507x 141x 4 3 2 7 4 3 2 9 8 7 4 3 2 5 4 3 2 9 8 7 6 5 4 3 2 1521x 81x AB’ (2x 14x x 7)( 3x 18x 3) 6x 36x 6x 42x 252x 42x 3x 18x 3x 21x 126x 21 6x 36x 6x 42x 255x 24x 3x 21x 126x 21 y’ A’B A 9 8 7 6 5 4 3 2 9 8 7 6 5 4 3 2 9 8 7 6 5 4 3 2 B’ 14x 126x 42x 322x 507x 141x 1521x 81x 6x 36x 6x 42x 255x 24x 3x 21x 126x 21 20x 162x 48x 280x 762x 165x 1524x 60x 126x 21 buluruz. www.matematikkolay.net 146
4 3 2 4 y (3x 4)(2x x 1) ise y’ ? 4 3 2 4 4 3 2 4 4 3 2 y (3x 4)(2x x 1) y’ (3x 4)'(2x x 1) (3x 4).4(2x x 1 : Çözüm 3 3 2 3 3 2 4 4 3 2 3 2 3 3 2 4 4 3 2 3 2 3 2 3 2 3 2 ) .(2x x 1)’ y’ 12x (2x x 1) (3x 4).4(2x x 1) .(6x 2x) y’ 12x (2x x 1) (3x 4).4(2x x 1) .(6x 2x) y’ 4x(2x x 1) 3x (2x x 1 4 3 2 3 5 4 2 5 4 3 2 3 5 4 2 ) (3x 4)(6x 2) y’ 4x(2x x 1) 6x 3x 3x 18x 6x 24x 8 y’ 4x(2x x 1) (24x 9x 3x 24x 8) buluruz. 147
4 3 3 2 y (x 5) (2x 1) ise y’ ? 4 3 3 2 4 2 4 3 2 4 3 3 3 3 4 y (x 5) (2x 1) y’ 3(x 5) (x 5)'(2x 1) (x 5) .2.(2x 1)(2x 1)’ y’ 12x (x 5 : ) Çözüm 2 3 2 2 4 3 3 2 3 4 2 3 4 2 3 4 2 4 4 2 3 4 2 4 2 3 4 2 4 (2x 1) 12x (x 5) (2x 1) y’ 12x (2x 1)(x 5) x(2x 1) (x 5) y’ 12x (2x 1)(x 5) 2x x x 5 y’ 12x (2x 1)(x 5) 3x x 5 y’ 12x (2x 1)(x 5) (3x x 5) buluruz. www.matematikkolay.net 148
4 2 (2x 1)(x 1) y ise y’ ? (x 2) A 4 2 B 4 2 6 4 2 5 3 2 (2x 1)(x 1) y (x 2) A (2x 1)(x 1) 2x 2x x 1 A’ 12x 8x 2x B x 2 B’ 1 A’B AB’ B : y’ Çözüm 5 3 6 4 2 2 6 5 4 3 2 6 4 2 2 6 5 4 3 2 6 4 2 2 6 5 4 3 2 2 (12x 8x 2x)(x 2) (2x 2x x 1).1 (x 2) 12x 24x 8x 16x 2x 4x (2x 2x x 1) (x 2) 12x 24x 8x 16x 2x 4x 2x 2x x 1 (x 2) 10x 24x 6x 16x x 4x 1 buluruz. (x 2) 149
3 (2x 1) (x 1) y ise y’ ? x 2 www.matematikkolay.net A 3 B 3 2 3 2 (2x 1) (x 1) y (x 2) A (2x 1) (x 1) A’ 3(2x 1) .2.(x 1) (2x 1) (2x 1) 6(x 1) (2x 1 : Çözüm 2 2 2 3 2 2 2 3 2 2 2 2 ) (2x 1) 6x 6 2x 1 (2x 1) (8x 5) A’B AB’ (2x 1) (8x 5)(x 2) (2x 1) (x 1).1 y’ B (x 2) (2x 1) (8x 5)(x 2) (2x 1) (x 1).1 (x 2) (2x 1) (8x 5)(x 2) (2x 1)(x 1) (x 2) (2x 1) (8x 5)(x 2) (2x 1)(x 1) (x 2 2 2 2 2 2 2 2 2) (2x 1) 8x 21x 10 2x x 1 (x 2) (2x 1) (6x 20x 11) buluruz. (x 2) 150

